Question

The law of refraction \(\frac{sin\,i}{sin\,r}=\frac{V_1}{V_2}\)

1. This law is called

2. Prove this law based on Huygiens wave theory.

Answer 1

1. Snells law

2. Refraction of a plane wave. (To prove Snell’s law): 

AB is the incident wavefront and c₁ is the velocity of the wavefront in the first medium. CD is the refracted wavefront and c₂ is the velocity of the wavefront in the second medium. AC is a plane separating the two media.

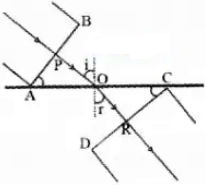
The time taken for the ray to travel from P to R is

O is an arbitrary point. Hence AO is a variable. But the time to travel a wavefront from AB to CD is constant. In order to satisfy this condition, the term containing AO in eq. (2) should be zero.

∴ \(AO\Big(\frac{sin\,i}{C_1}-\frac{sin\,r}{C_2}\Big)\) = 0

\(\frac{sin\,i}{C_1}=\frac{sin\,r}{C_2}\)

\(\frac{sin\,i}{sin\,r}=\frac{C_1}{C_2}\) = ¹n₂(constant)

where ¹n₂ is the refractive index of the second medium w.r.t. the first. This is the law of refraction.