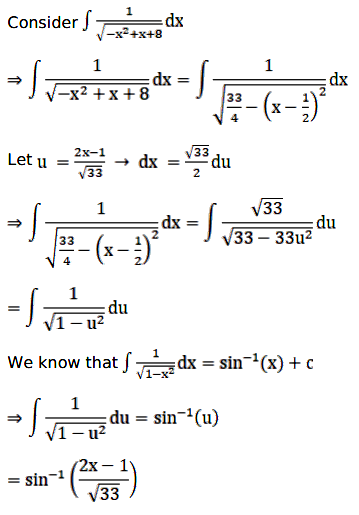Evaluate the following integrals : ∫x/√(8+x-x^2)dx

Question

Evaluate the following integrals :

\(\int\frac{x}{\sqrt{8+x-x^2}}\)dx

Answer 1

Given,

I = \(\int\frac{x}{\sqrt{-x^2+x+8}}\)dx

 Integral is of form

\(\int\frac{px+q}{\sqrt{ax^2+bx+c}}\)dx

Writing numerator as,

 px + q = λ \(\{\frac{d}{dx}(ax^2+bx+c)\}+μ \)

⇒ px + q = λ(2ax + b) + μ 

⇒ x = λ (-2x + 1) + μ 

∴ λ = -1/2 and μ = -1/2 

Let x = -1/2(-2x + 1) – 1/2 and split,