Question

Two rods A and B of same length and cross-sectional area are connected in series and a temperature difference of `100^@C` is maintained across the combination as shoen in Fig. If the thermal conductivity of the rod A is 3 k and that of rod B is k, Then
i.Determine the thermal resistance of each rod.
ii. determine the heat current flowing through each rod.
iii. determine the heat current flowing through each rod.
iv. plot the variation of temperature along the length of the rod.

Answer 1

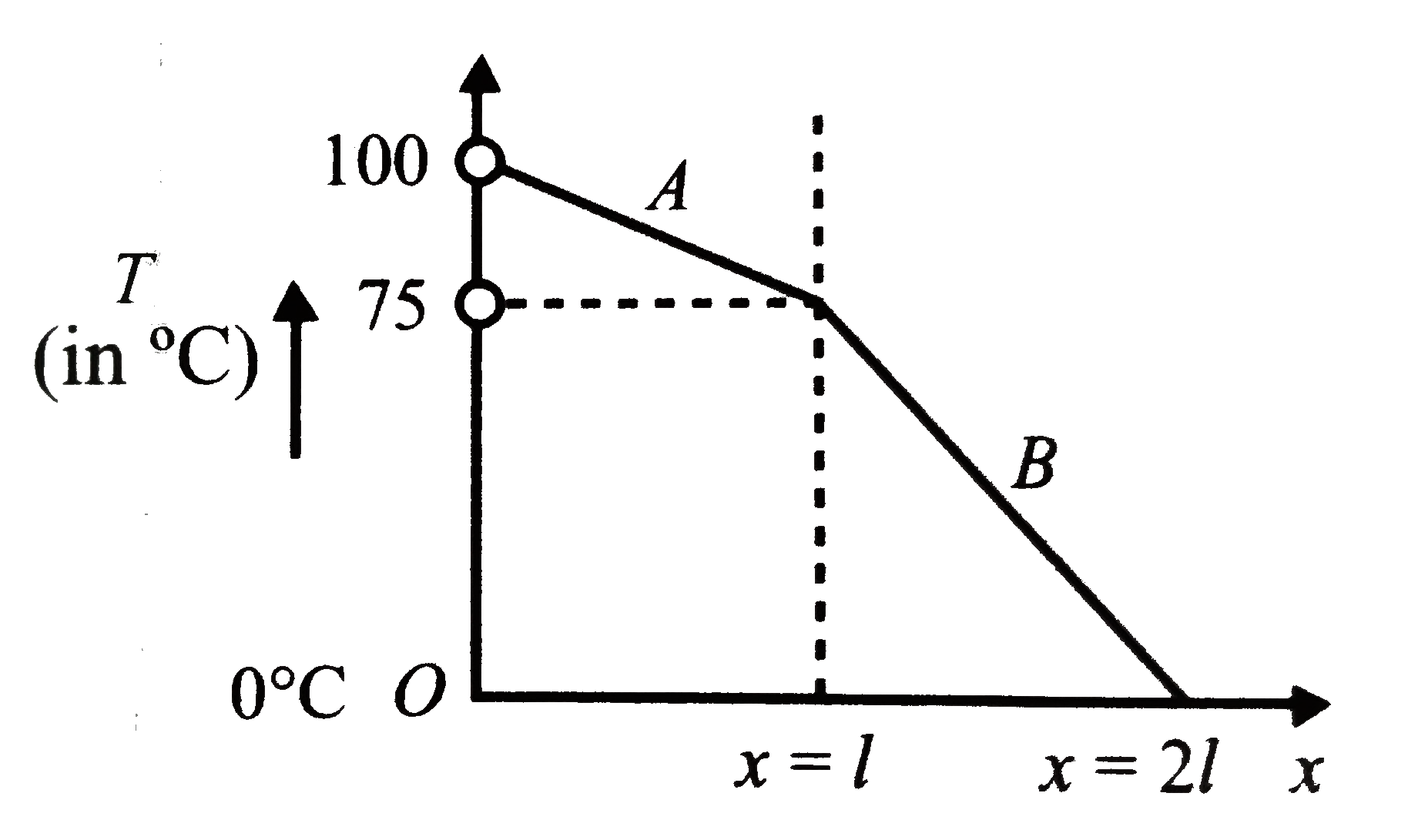
i. Thermal resistance is defined as `R=l//kA`
For rod A `R_A=(l)/(k_(A)A)=(l)/(3kA)`
For rod B `R_B=(l)/(k_(B)A)=(l)/(kA)`
ii. Using electrical analogy, the given problem may be modified as The equivalent resistance is `R=R_A+R_B=(4)/(3)(l)/(kA)`
The heat current is given by
`I=(T_1-T_2)/(R )=(100-0)/((4)/(3)(l)/(kA))=73((kA)/(l))`
iii. The temperature of the junction is given by
`T=T_1-IR_A=100-(75kA)/(l)((l)/(3kA))=75^@C`
iv. The variation of temperature along the length of the conductor is shown in Fig.