Question

A river `500 m` wide flows at a rate at a rate of `4 km h^(-1)`. A swimmer who can swim at `8 km h^(-1)`. In still water, wishes to cross the river straight. (i) Along what direction must he strike? (ii) What should be his resultant velocity. (iii) What is the time of crossing the river?

Answer 1

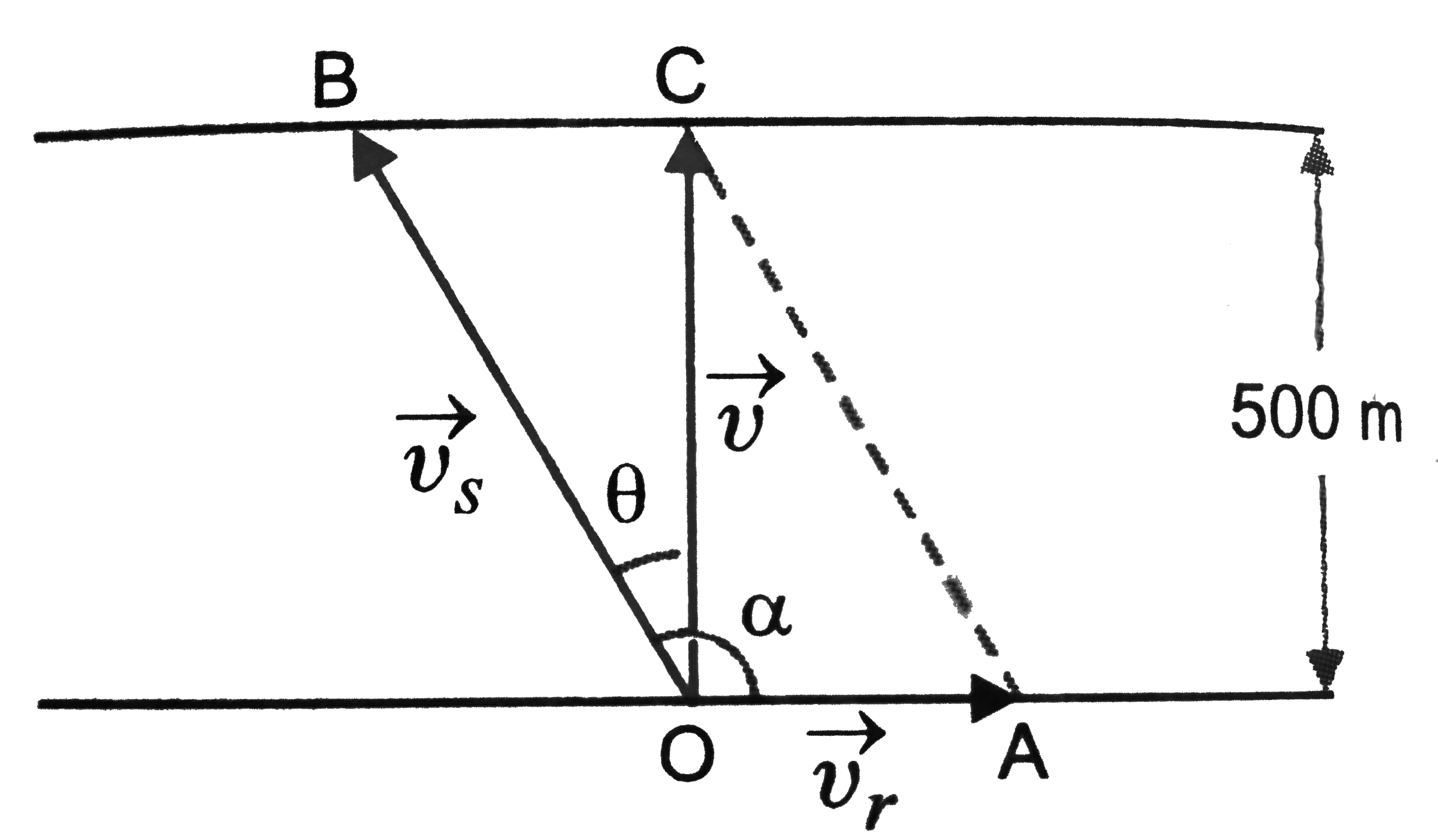
(i) Refer to fig. 2(c ) 22,
velocity of river `=4 km h^(-1) =(OA)`
Velcity of swinmmer in still water
`v_(s0 =8 km h^(-10 =(OB)`
The swimmer will cross the river straitght if the resultant velocity `vec v of vec v_(r) and vec v_(s) ` is perpendiculat to the bank fof the river, i.e., along `(vec OC)`. This will be possible if the swimmer groes upatream of the river along `OB`, making an angle `theta` with `OC`. In right `DeltaOCB`, sin `theta (NC)/(OB) =v_(r)/v_(s0 =4/8 =1/2 =sin 30^(00`
:. `theta =30^(00` (ii) Resultant velocity of the swimmer
`v= sqrt(v_(s)^(2) -v_(r)^(2)) =sqrt (8^(20-4^(2)) =sqrt 48 =4 sqrt 3 km h^(-1) =4 sqrt3 xx 5// 18 =1.92 m//s
(iii) Time taken to cross the river, `t=(width of river)/v =(500 m)/(1.92 ms^(-1)) =260.4 s`.
.