Question

A unifrom cube of mass `m` and side a is placed on a frictionless horizontal surface. A vertical force `F` is applied to the edge as shown in Fig. Match the following (most appropriate choice) :
(a)`mg//4 lt F lt mg//2` (i) Cube will move up.
(b) `F gt mg//2` (ii) Cube will not exhibit motion.
(c ) `F gt mg` (iii) Cube will begin to rotate and slip at `A`.
(d) `F = mg//4` (iv) Normal reaction effectively at `a//3` from A, no motion.

Answer 1

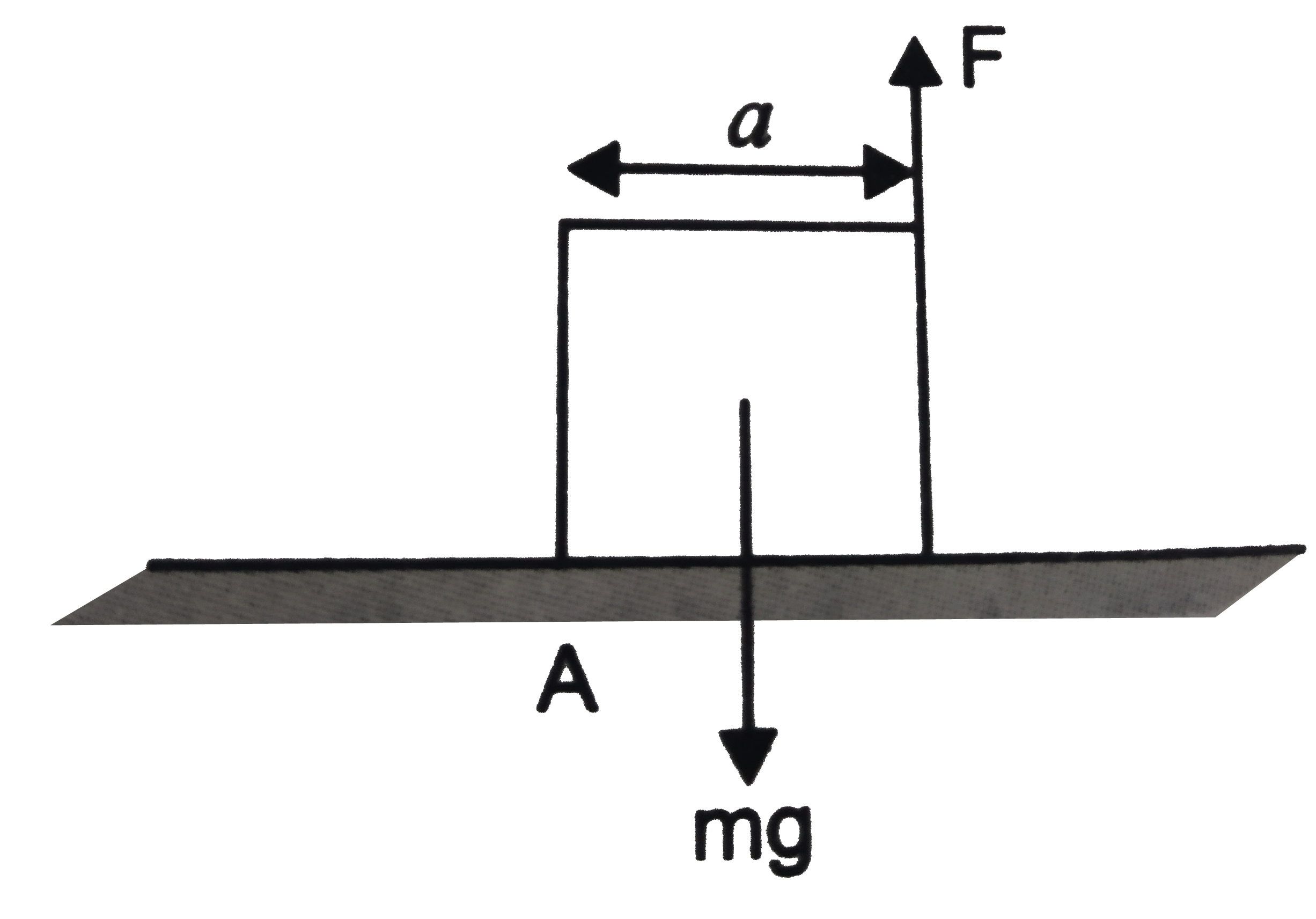
As is clear form Fig. torque of force `F` about `A, tau_(1) = F xx a`. This is anticlockwise.
Torque of weight `mg` of cube about `A`
`tau_(2) = mg xx (a)/(2)`, clockwise. The cube will not exhibit any motion if `tau_(1) = tau_(2)`
`F xx a = mg xx (a)/(2) or F = (mg)/(2)`
The cube will rotate only when `tau_(1) gt tau_(2)`
`F xx agt mg.(a)/(2) or F gt (mg)/(2)`
If we assume that normal reaction is effectively at `a//3` from `A`, then block would turn if
`mg xx (a)/(3) = F xx a or F = (mg)/(3)`
When `F = (mg)/(4) lt (mg)/(3)`, there will be no motion.
Hence, we conclude (a) rarr (ii), (b) rarr (iii), (c ) rarr (i), (d) rarr (iv).