Question

A diet of a sick person must contains at least 48 units of vitamin A and 64 units of vitamin B. Two foods `F_(1) and F_(2)` are available. Food `F_(1) ` costs Rs. 6 per unit and Food `F_(2) ` costs Rs. 10 per unit. One unit of food `F_(1) ` contains 6 units of vitamin A and 7 units of vitamin B. One unit of food `F_(2)` contains 8 units of vitamin A and 12 units of vitamin B. Find the minimum cost for the diet that consists of mixture of these two foods and also meeting the minimum nutritional requirements.

Answer 1

Let x units of food `F_(1)` and y units of food `F_(2)` be included in the diet of the sick person.
Then their total cost is Z Rs. `(6x + 10y)`
We have to minimize the above cost function . The constraints are as per given the following table.
`{:(,"Food "F_(1),"Food "F_(2),"Minimum"),(,(x),(y),"requirement"),("Vitamin A",6,8," "48),("Vitamin C",7,12," "64):}`
Hence the constraints are
` 6x + 8y ge 48`
` 7x + 12y ge 64`
Also the no. of units of food ` F_(1) and F_(2)` cannot be negative.
` x ge 0 and y ge 0`
Hence the mathematical formulation of given LPP is
Minimize `Z = 6x + 10y`
Subject to ` 6x + 8y ge 48`
` 7x + 12y ge 64`
` rArr x/(64//7) + y/(16/3) = 1`
Plot these equations on graph paper we get feasible region shaded on graph paper.

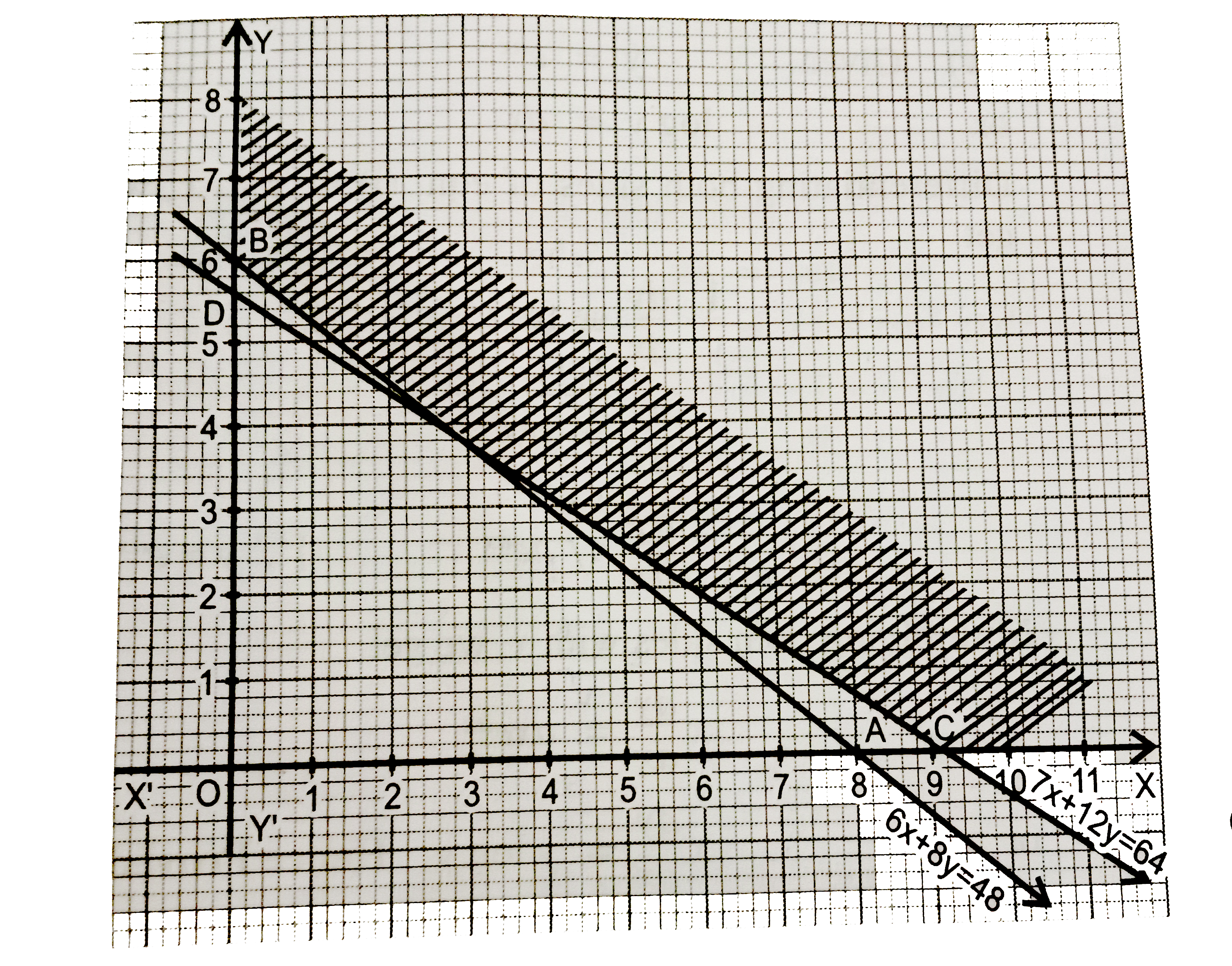
The vertices of the feasible region are ` C(64/7, 0), P ` and B (0, 6).
P is the point of intersection of these lines
` 6x + 8y = 48`
and ` 7x + 12y = 64`
On solving these equations we get `P-=(4,3)` . Values of objective function at these vertices are
` Z(C) = 6 (64/7) + 10 (0) = (384)/7`
` = 54*85`
` Z(P) = 6(4) + 10(3) 24 + 30 = 54`
` Z(B) = 6(0)+10(6) = 60`
Minimum value of Z is 54 at point P(4,3) hence, 4 units of food `F_(1) and 3` units of food `F_(2)` should be included in the diet of sick person to meet the minimal nutritional requirements, in order to have the minimum cost of Rs. 54.