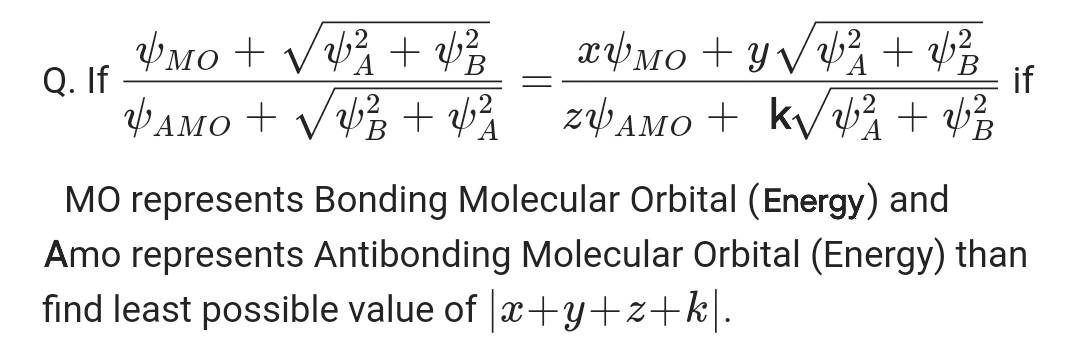
Q. If \( \frac{\psi_{M O}+\sqrt{\psi_{A}^{2}+\psi_{B}^{2}}}{\psi_{A M O}+\sqrt{\psi_{B}^{2}+\psi_{A}^{2}}}=\frac{x \psi_{M O}+y \sqrt{\psi_{A}^{2}+\psi_{B}^{2}}}{z \psi_{A M O}+ k \sqrt{\psi_{A}^{2}+\psi_{B}^{2}}} \) if
MO represents Bonding Molecular Orbital (Energy) and Amo represents Antibonding Molecular Orbital (Energy) than find least possible value of \( |x+y+z+k| \).