Question

A unifrom solid disk of radius `R` and mass `M` is free to rotate on a frictionless pivot through a point on its rim. If the disk is released from rest in the position shown in figure. The speed of the lowest point on the disk in the dashed position is.
.
A. `4 sqrt((Rg)/(3))`
B. `2 sqrt((Rg)/(3))`
C. `sqrt((Rg)/(3))`
D. `3 sqrt((Rg)/(2))`

Answer 1

Correct Answer - A
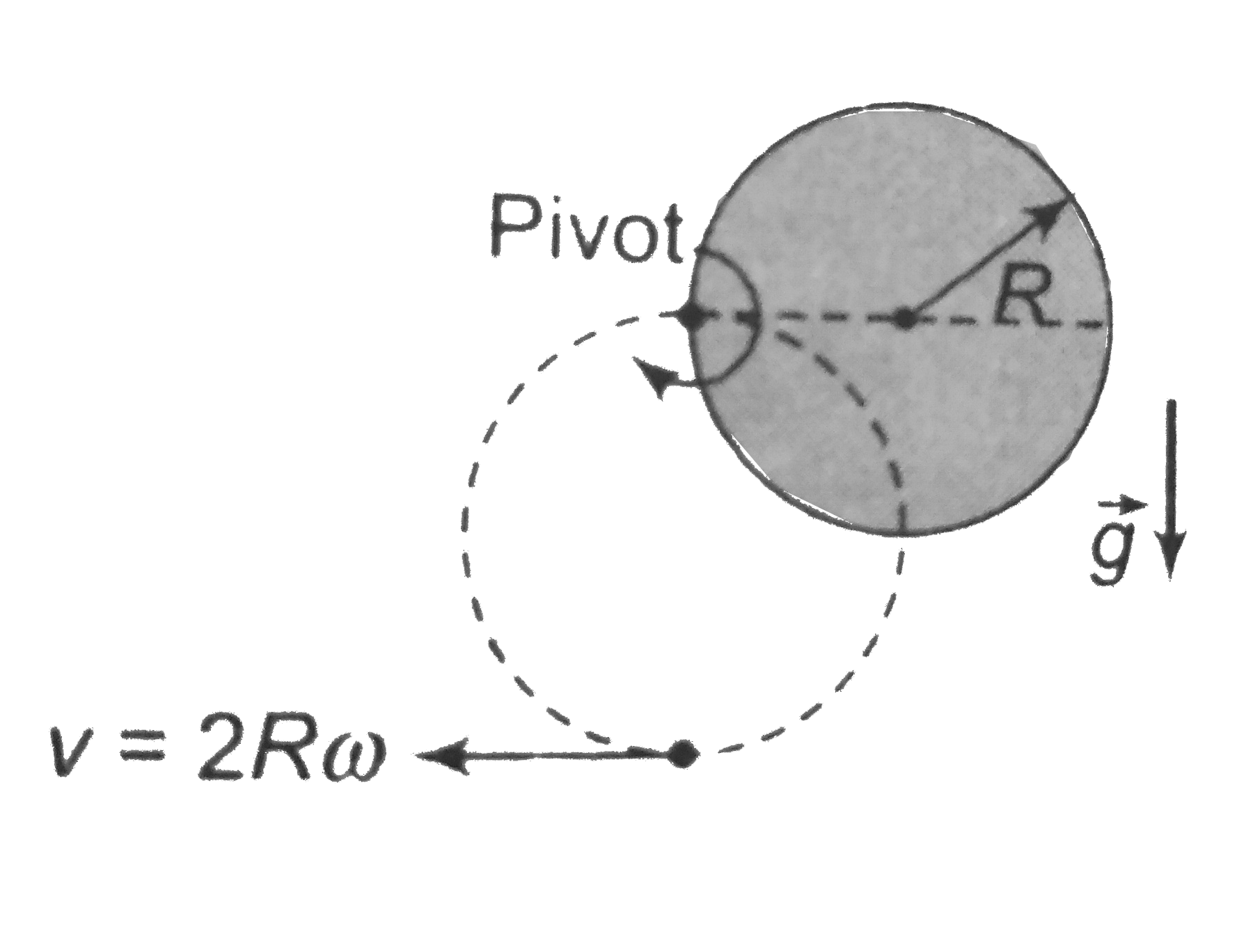
(a) To identify the change in gravitational energy, think of the height through which the centre of mass falls. From the parallel-axis theorem, the moment of inertia of the disk about the pivot point on the circumference is
`I = I_(CM) + MD^2 = (1)/(2) MR^2 + MR^2 = (3)/(2) MR^2`
The pivot point is fixed, so the kinetic energy is entirely rotational around the pivot. The equation for the isolated system (energy) model
`(K + U_f) = (K + U)_f`
for the disk-Earth system becomes
`0 + MgR = (1)/(2) ((3)/(2) MR^2) omega^2 + 0`
Solving for `omega`, `omega= sqrt((4 g)/(3 R))`
At the lowest point on the rim, `v = 2 R omega = 4 sqrt((R g)/(3))`.
.