Question

NCERT Solutions Class 12 Physics Chapter 1 Electric Charges and Fields is a very important chapter of class 12. One must go through our detailed NCERT Solutions for complete preparation for the CBSE board exams.

NCERT Solutions Class 12 is based on the latest syllabus proposed by the CBSE. It is designed in a detailed manner and all the important concepts are discussed here such as:

• Electric charges and fields – the electric charge are of two kinds namely positive and negative charges. Like charges repel each other while the unlike charges attract each other. The positive charge is mainly carried by the positive part of the atom called the proton and negative charges are carried by the negative part called the electron. When there is an unequal number of positive and negative charges in an atom then the atom is called an ion. For bigger objects, we have a negative charge that means more amount of electrons while a positive charge is called fewer electrons.
• Conductors – conductors are those materials that allow the flow of electrons very easily and are called conductors. Examples of conductors are metals, electrolytes, superconductors, semiconductors, plasmas, and some non-metallic substances like graphite. The conduction band of some of the metallic conductors is silver 1.59 * 10^-8 to 6.30*10⁷.
• Insulators – insulators are materials that do not conduct electricity. Example of insulators is wood, plastic, clothes, pure water, etc.
• Charging by induction – the process of charging an object without touching it is called charging by induction.
• Coulomb’s Law – according to the coulombs law the force of attraction or the repulsion between two charges is directly proportional to the product of their charges and inversely proportional to the length of the distance between them.

NCERT Solutions Class 12 Physics will help students learn and revise every concept thoroughly. The solution provided here has detailed answers to all kinds of queries.

Answer 1

NCERT Solutions Class 12 Physics Chapter 1 Electric Charges and Fields

1. What is the force between two small charged spheres having charges of 2 × 10^–7C and 3 × 10^–7 C placed 30 cm apart in the air?

Answer:

Given,

The Charge on the 1^st sphere and 2^nd sphere is q₁ = 2 x 10^-7 C and q₂ = 3 x 10^-7 C

The distance between two charges is given by r = 30cm = 0.3m

The electrostatic force between the spheres is given by the relation :

\(F = \frac{1}{4\pi\epsilon_0}.\frac{q_1q_2}{r^2}\)

Here,

ϵ_0​ = permittivity of free space and,

\(\frac{1}{4\pi\epsilon_0} = 9 \times10^9\,Nm^2C^{-2}\)

Force, 

\(F = \frac{9 \times10^9\times2\times10^{-7}\times3\times10^{-7}}{(0.3)^2}= 6\times10^{-3}N.\) 

The force between the charges will be repulsive as they have the same nature.

2. The electrostatic force on a small sphere of charge 0.4 µC due to another small sphere of charge –0.8 µC in the air is 0.2 N.

(a) What is the distance between the two spheres?

(b) What is the force on the second sphere due to the first?

Answer:

(a) Given,

The charge on 1^st sphere (q₁) and 2^nd sphere (q₂) is 0.4 µC or 0.4 × 10^-6 C and -0.8 × 10^-6C respectively.

The electrostatic force on the 1^st sphere is given by F = 0.2N.

Electrostatic force between the spheres is given by the relation:

\(F = \frac{1}{4\pi\epsilon_0}.\frac{q_1q_2}{r^2}\)

Here,

ϵ_0​ = permittivity of free space and,

\(\frac{1}{4\pi\epsilon_0} = 9 \times10^9\,Nm^2C^{-2}\)

\(r^2= \frac{1}{4\pi\epsilon_0}.\frac{q_1q_2}{F}\)

\(= \frac{0.4 \times 10^{-6}\times8 \times10^{-6}\times9 \times 10^{-9}}{0.2}= 144\times10^{-4}\)

\(r = \sqrt{144\times 10^{-4}} = 12 \times 10^{-2} = 0.12 \,m\)

Therefore, the distance between the two spheres = 0.12 m

(b) Since the spheres have opposite charges, the force on the second sphere due to the first sphere will also be equal to 0.2N.

3. Check that the ratio ke²/G m_em_p is dimensionless. Look up a Table of Physical Constants and determine the value of this ratio. What does the ratio signify?

Answer:

The ratio to be determined is given as follows :

\(\frac{ke^2}{Gm_em_p}\)

where G is the gravitational constant in N m² kg^-2

m_e and m_p is the masses of electron and proton in kg.

e is the electric charge (unit – C)

\(k = \frac{1}{4\pi\epsilon_0}\)   (unit – Nm²C^-2)

Therefore, the unit of given ratio,

\(\frac{ke^2}{Gm_em_p}=\frac{|Nm^2C^{-2}||C^{-2}|}{|Nm^2kg^{-2}||kg||kg|}=M^0L^0T^0\)

So, the given ratio is dimensionless.

Given,

e = 1.6 x 10^-19 C

G = 6.67 x 10^-11 N m² kg^-2

m_e = 9.1 x 10^-31 kg

m_p = 1.66 x 10^-27 kg

Putting the above values in the given ratio, we get

\(\frac{ke^2}{Gm_em_p}=\frac{9\times10^9\times(1.6\times10^{-19})^2}{6.67 \times 10^{-11}\times9.1\times10^{-31}\times1.67\times10^{-27}}= 2.3 \times 10^{39}\)

So, the above ratio is the ratio of the electric force to the gravitational force between a proton and an electron when the distance between them is constant.

4. (i) Explain the meaning of the statement ‘electric charge of a body is quantised’.

(ii) Why can one ignore quantisation of electric charge when dealing with macroscopic i.e., large scale charges?

Answer:

(i) The ‘electric charge of a body is quantized’ means that only integral (1, 2, …n)  numbers of electrons can be transferred from a body to another.

Charges cannot get transferred in fractions. Hence, the total charge possessed by a body is only in integral multiples of electric charge.

(ii) In the case of large scale or macroscopic charges, the charge which is used over there is comparatively too huge to the magnitude of the electric charge. Hence, on a macroscopic level, the quantization of charge is of no use Therefore, it is ignored and the electric charge is considered to be continuous.

5. When a glass rod is rubbed with a silk cloth, charges appear on both. A similar phenomenon is observed with many other pairs of bodies. Explain how this observation is consistent with the law of conservation of charge.

Answer:

When two bodies are rubbed against each other,  a charge is developed on both bodies. These charges are equal but opposite in nature. And this phenomenon of inducing a charge is known as charging by friction. The net charge on both of the bodies is 0 and the reason behind it is that an equal amount of charge repels it. When we rub a glass rod with a silk cloth, charge with opposite magnitude is generated over there. This phenomenon is in consistence with the law of conservation of energy. A similar phenomenon is observed with many other pairs of bodies.

6. Four point charges q_A​ = 2μC, q_B​= −5μC, q_C​ = 2μC and q_D​= −5μC are located at the corners of a square ABCD of side 10 cm. What is the force on a charge of 1 µC placed at the centre of the square?

Answer:

In the above picture, we have shown the square mentioned in the question. Whose side is 10 cm and four charges are placed at the corners of the squares And O is the centre of the square.

Where,

(Sides) AB = BC = CD = DA = 10 cm

(Diagonals) AC = BD = 10√2​ cm

AO = OC = DO = OB = 5√2 ​cm

At the centre point ‘O’, we have placed a charge of 1 μC

In the above case, the repulsive force between the corner A and the centre O is same in magnitude with the repulsive force by the corner C to the centre O, but these forces are opposite in direction. Hence, these forces will cancel each other and from A and C no forces are applied on the centre O. Similarly, from the corner C the attractive force is applying on to the centre O and another force with the same magnitude is applying on the centre O, also these two forces are opposite in direction hence they are also opposing each other.

Therefore, the net force applying to the centre is zero. Because all the forces here are being cancelled by each other.

7. (i) An electrostatic field line is a continuous curve. That is, a field line cannot have sudden breaks. Why not?

(ii) Explain why two field lines never cross each other at any point.

Answer:

(i) When a charge is placed in an electrostatic field then it experiences a continuous force. Therefore, an electrostatic field line is a continuous curve. And a charge moves continuously and does not jump from on point to the other. So, the field line cannot have a sudden break.

(ii) if two field lines will cross each other at any point then at that point the field intensity will start shooing two directions at the same point which is impossible. Therefore, two field lines can never cross each other.

Answer 2

8. Two point charges q_A = 3 µC and q_B= –3 µC are located 20 cm apart in a vacuum.

(i) What is the electric field at the midpoint O of the line AB joining the two charges?

(ii) If a negative test charge of magnitude 1.5 × 10^–9 C is placed at this point, what is the force experienced by the test charge?

Answer:

(i) The figure given below shows the situation given to us, in which AB is a line and O is the midpoint.

Distance between two charges, AB = 20 cm

Therefore, AO = OB = 10 cm

Total electric field at the centre is (Point O) = E

Electric field at point O caused by +3 μC charge,

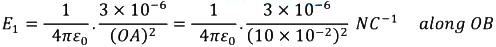
Where ϵ₀​ = Permittivity of free space and \(\frac1{4\pi\epsilon_0} \) = 9 × 10⁹ Nm²C⁻²

Therefore,

Electric field at point O caused by −3 μC charge,

[Since the magnitudes of E₁​ and E₂​ are equal and in the same direction]

Therefore, the electric field at mid – point O is 5.4 × 10⁶ NC⁻¹ along OB.

(ii) A test charge with a charge potential of 1.5 × 10⁻⁹C is placed at mid – point O.

q = 1.5 × 10⁻⁹ C

Let the force experienced by the test charge be  F

Therefore, F = qE

= 1.5 × 10−9 × 5.4 × 10⁶

= 8.1 × 10⁻³N

The force is directed along line OA because the negative test charge is attracted towards point A and is repelled by the charge placed at point B. As a result, the force experienced by the test charge is q = 8.1 × 10⁻³ N along OA.

9. A system has two charges q_A​ = 2.5 × 10⁻⁷ C and q_B​= −2.5 × 10⁻⁷C located at points A :(0, 0, -15 cm) and B (0, 0, + 15 cm), respectively. What is the total charge and electric dipole moment of the system?

Answer:

The charges which are located at the given points are shown in the co-ordinate system as:

At point A, total charge amount, q_A ​= 2.5 × 10⁻⁷ C

At point B, total charge amount, q_B​ = –2.5 × 10⁻⁷ C

Total charge of the system is, q_A ​+ q_B​ = 2.5 × 10⁻⁷ C –2.5 × 10⁻⁷C=0

Distance between two charges at points A and B,

d = 15 + 15 = 30 cm = 0.3 m

Electric dipole moment of the system is given by,

p=q_A​ × d = q_B​ × d = 2.5 × 10⁻⁷ × 0.3

=7.5 × 10⁻⁸ C m along positive z – axis

Therefore, the electric dipole moment of the system is 7.5 × 10⁻⁸ C m along positive z−axis.

10. An electric dipole with dipole moment 4 × 10⁻⁹ Cm is aligned at 30° with the direction of a uniform electric field of magnitude 5 × 10⁴ NC⁻¹. Calculate the magnitude of the torque acting on the dipole.

Answer:

Electric dipole moment, p = 4 × 10⁻⁹Cm

Angle made by p with a uniform electric field, θ = 30^∘

Electric field, E = 5 × 10⁴ NC⁻¹

Torque acting on the dipole is given by the relation,

τ = pEsinθ

= 4 × 10⁻⁹ × 5 × 10⁴ × sin30 = 20 × 10⁻⁵ × \(\frac12\)=10⁻⁴ Nm

Therefore, the magnitude of the torque acting on the dipole is 10⁻⁴Nm.

11. A polythene piece rubbed with wool is found to have a negative charge of 3 × 10⁻⁷C.

(i) Estimate the number of electrons transferred (from which to which?)

(ii) Is there a transfer of mass from wool to polythene?

Answer:

(i) Since the wool is positively charged and the polythene is negatively charged, so we can say that few amounts of electrons are transferred from wool to polythene.

Charge on the polythene, q = 3 × 10⁻⁷C.

Amount of charge on an electron, e =−1.6 × 10⁻¹⁹C

Let number of electrons transferred from wool to polythene be n

So, by using the given equation we can calculate the value of n,

q = ne

⇒ \(n = \frac qe = \frac{-3 \times10^{-7}}{-1.6\times10^{-19}}= 1.87 \times 10^{12}\)

Therefore, the number of electrons transferred from wool to polythene is 1.87 × 10¹²

(ii) Yes,

Mass is also transferred as an electron is transferred from wool to polythene and an electron particle have some mass.

Mass of an electron, m_e​ = 9.1 × 10⁻³¹ kg

Total mass transferred , m = m_e​ × n

= 9.1 × 10⁻³¹ × 1.87 × 10¹²

= 1.701 × 10⁻¹⁸ kg

Here, the mass transferred is too low that it can be neglected.

12. (i) Two insulated charged copper spheres A and B have their centres separated by a distance of 50 cm. What is the mutual force of electrostatic repulsion if the charge on each is 6.5 × 10⁻⁷C each? The radii of A and B are negligible compared to the distance of separation.

(ii) What is the force of repulsion if each sphere is charged double the above amount, and the distance between them is halved?

Answer:

(i) Charge on sphere A, q_A​ = 6.5 × 10⁻⁷C

Charge on sphere B, q_B ​= 6.5 × 10⁻⁷C

Distance between the spheres, r = 50 cm = 0.5 m

Force of repulsion between the two spheres,  

\(F = \frac{1}{4\pi\epsilon_0}.\frac{q_Aq_B}{r^2}\)

Where ϵ₀​ = Permittivity of free space and \(\frac{1}{4\pi\epsilon_0} = 9 \times 10^9 Nm^2C^2\)

Therefore,

\(F = \frac{9\times10^9\times(6.5\times 10^{-7})^2}{(0.5)^2}\)

= 1.52 × 10⁻²N

Therefore, the force between the two spheres is 1.52 × 10⁻² N

(ii) After doubling the charge,

Charge on sphere A, q_A​ = 1.3 × 10⁻⁶C

Charge on sphere B, q_B​ = 1.3 × 10⁻⁶C

The distance between the spheres is halved.

\(\therefore r = \frac{0.5}{2} = 0.25\, m\)

Force of repulsion between the two spheres,

\(F = \frac{1}{4\pi\epsilon_0}.\frac{q_Aq_B}{r^2} \)

\(= \frac{9\times 10^9\times1.3\times10^{-6}\times 1.3 \times10^{-6}}{(0.25)^2}\)

= 16 × 1.52 × 10⁻²

= 0.243N

Therefore, the force between the two spheres is 0.243 N.

Answer 3

13. Suppose the spheres A and B in Exercise 1.12 have identical sizes. A third sphere of the same size but uncharged is brought in contact with the first, then brought in contact with the second, and finally removed from both. What is the new force of repulsion between A and B?

Answer:

Distance between the spheres, A and B, r = 0.5 m

Initially, the charge on each of sphere q = 6.5 × 10⁻⁷C

When the sphere A is touched with An uncharged sphere C, then half of the charge will be transferred to the sphere C. Hence the charge on both the spheres A and C will be q/2.

After that when sphere C with charge q/2 is brought in touch with sphere B with charge q, then charge on each of the sphere will be divided in two equal parts, is.

\(\frac12(q +\frac q2) = \frac{3q}{4}\)

Hence, charge on each of the spheres, C and B , is \(\frac{3q}{4}\)

Force of repulsion between sphere A and B is:

\(F = \frac{1}{4\pi\epsilon_0}.\frac{q_Aq_B}{r^2} \)

\(=\cfrac{1}{4\pi\epsilon_0}.\cfrac{\frac q2\times\frac{3q}4}{r^2}\)

\( = \frac{1}{4\pi\epsilon_0}.\frac{3q^2}{8r^2} \)

\(= \frac{9 \times10^9\times3\times(6.5\times10^{-7})^2}{8\times(0.5)^2}\)

\( = 5.703\times10^{-3}N\)

Therefore, the force of attraction between the two spheres is 5.703 × 10⁻³ N

14. The figure below shows tracks of three charged particles in a uniform electrostatic field. Give the signs of the three charges. Which particle has the highest charge to mass ratio?

Answer:

We can see here that the particles 1 and 2 are moving in the direction of the positive charge and we know that the opposite charge attracts each other and the same charge repels each other. So, here we can say that the charged particles 1 and 2 which are going towards the charged particle are negatively charged. And the particle 3 is being attracted towards the negative charge. So, the particle 3 will be the positively charged particle.

The EMF or charge to mass ratio is directly proportional to the amount of depletion or depletion at a given velocity. Here we can see that the particle 3 is depleting more as compared to the other two. Therefore, it will have a higher charge to mass ratio.

15. Consider a uniform electric field E = 3 × 10 ³ î N/C.

(a) What is the flux of this field through a square of 10 cm on a side whose plane is parallel to the y z – plane?

(b) What is the flux through the same square if the normal to its plane makes a 60° angle with the x-axis?

Answer:

(a) Electric field intensity, E = 3 × 10³ î  N / C

Magnitude of electric field intensity, | E | = 3 × 10³ N / C

Side of the square,  s = 10 cm = 0.1 m

Area of the square, A = s² = 0.01 m²

The plane of the square is parallel to the y – z plane. Hence, angle between the unit vector normal to the plane and electric field, θ = 0°

Flux ( φ ) through the plane is given by the relation,

φ = |E|A cos θ

φ  = 3 × 10³ × 0.01 × cos 0°

φ = 30 N m² /C

(b) Plane makes an angle of 60° with the x – axis.

Hence, θ = 60°

Flux, φ = | E |A cos θ

Flux, φ  = 3 × 10³ × 0.01 × cos 60°

Flux, φ  = 30 x 0.5

Flux, φ  = 15 N m² /C

16. What is the net flux of the uniform electric field of Exercise 1.15 through a cube of side 20 cm oriented so that its faces are parallel to the coordinate planes?

Answer:

All the faces of a cube are parallel to the coordinate axes. Therefore, the number of field lines entering the cube is equal to the number of field lines piercing out of the cube. As a result, net flux through the cube is zero.

17. Careful measurement of the electric field at the surface of a black box indicates that the net outward flux through the surface of the box is 8.0 × 10³ N m² /C.

(a) What is the net charge inside the box?

(b) If the net outward flux through the surface of the box were zero, could you conclude that there were no charges inside the box? Why or Why not?

Answer:

(a) Net outward flux through the surface of the box, φ = 8.0 × 10³ Nm² /C

For a body containing net charge q, flux is given by the relation,

\(\phi = \frac q{\epsilon_0}\)

ε_0​= permitivity of free space

ε₀​ = 8.854 × 10^–12 N^–1 C² m^–2

q = ε₀​ϕ

= 8.854 x 10^–12 x 8.0 x 10³ C = 7.08 x 10^–8 C = 0.07 μC

Thus, the total charge inside the box is 0.07 μC

(b) No

Net flux piercing out through a body depends on the net charge contained in the body. If net flux is zero, then it can be inferred that net charge inside the body is zero. The body may have equal amount of positive and negative charges.

18. A point charge + 10 μC is at a distance 5 cm directly above the centre of a square of side 10 cm, as shown in Fig. What is the magnitude of the electric flux through the square?

(Think of the square as one face of a cube with edge 10 cm)

Answer:

The square can be considered as one face of a cube of edge 10 cm with a centre where charge q is placed. According to Gauss’s theorem for a cube, total electric flux is through all its six faces.

\(\phi_{Total} = \frac q{\epsilon_0}\)

Hence, electric flux through one face of the cube i.e., through the square is

\(\phi = \frac{\phi_{Total}}{6} = \frac16.\frac {q}{\epsilon_0}\)

Here,

ϵ_0​ = permittivity of free space = 8.854 x 10^–12 N^–1 C² m^–2

q = 10μC = 10 × 10^–6C

Therefore,

\(\phi =\frac16.\frac{10\times10^{-6}}{8.854\times10^{-12}}\)

\(\phi\) = 1.88 x 10⁵ Nm² C^–1

Therefore, electric flux through the square is 1.88 × 10⁵ Nm² C^–1.

19. A point charge of 2.0 μC is at the centre of a cubic Gaussian surface 9.0 cm on edge. What is the net electric flux through the surface?

Answer:

Net electric flux (\(\phi\)_Net) through the cubic surface is given by

\(\phi_{net} = \frac q {\epsilon_0}\)

Here,

ϵ_0​ = permittivity of free space = 8.854 × 10^–12 N^–1 C² m^–2

q = total charge contained in the cube given = 2.0μC = 2 × 10^–6C

Therefore,

\(\phi_{net} = \frac{2 \times 10^{-6}}{8.854\times10^{-12}}\)

\(\phi_{net} = 2.26 \times 10^5 Nm^2 C^{-1}\)

The total electric flux through the surface of the cube given is, \(\phi_{net} = 2.26 \times 10^5 Nm^2 C^{-1}\)

Answer 4

20. A point charge causes an electric flux of – 1.0 × 10³ Nm²/C to pass through a spherical Gaussian surface of 10.0 cm radius centred on the charge.

(a) If the radius of the Gaussian surface were doubled, how much flux would pass through the surface?

(b) What is the value of the point charge?

Answer:

(a) Electric flux, Φ = – 1.0 × 10³ Nm²/C

Radius of the Gaussian surface, r = 10.0 cm

Electric flux penetrating through a surface depends on the net charge enclosed inside the body. It does not depend on the size of the body. If the radius of the Gaussian surface is doubled, then the flux passing through the surface remains the same i.e., – 10³ Nm²/C.

(b) Electric flux is given by the relation

\(\phi_{Total} = \frac q{\epsilon_0}\)

Here,

ϵ₀​ = permittivity of free space = 8.854 x 10^–12 N^–1 C² m^–2

q = total charge contained enclosed by the spherical surface = φ ε₀

q = – 1.0 x 10³ x 8.854 x 10^–12

q = – 8.854 x 10^–9 C

q = – 8.854 n C

Therefore, the value of the point charge is – 8.854 n C.

21. A conducting sphere of radius 10 cm has an unknown charge. If the electric field 20 cm from the centre of the sphere is 1.5 × 10³ N/C and points radially inward, what is the net charge on the sphere?

Answer:

Electric field intensity (E) at a distance (d) from the centre of a sphere containing net charge q is given by the relation,

\(E = \frac{1}{4\pi\epsilon_0}.\frac{q}{d^2}\)

Where, q = Net charge = 1.5 × 10 ³ N/C

d = Distance from the centre = 20 cm = 0.2 m

ε₀ = Permittivity of free space and \(\frac 1 {4\pi\epsilon_0} = 9 \times 10^9 Nm^2C^{-2}\)

Therefore, the net charge on the sphere is 6.67 n C.

22. A uniformly charged conducting sphere of 2.4 m diameter has a surface charge density of 80.0 μC /m ²

(a) Find the charge on the sphere.

(b) What is the total electric flux leaving the surface of the sphere?

Answer:

(a) The diameter of the sphere, d = 2.4 m

The radius of the sphere, r = 1.2 m

Surface charge density, σ = 80.0 μC /m² = 80 × 10^–6 C/m ²

The total charge on the surface of the sphere can be calculated as follows,

Q = Charge density × Surface area

= σ × 4πr²

= 80 × 10^–6 × 4 × 3.14 × ( 1.2 )²

= 1.447 × 10^–3 C

Therefore, the charge on the sphere is 1.447 × 10^–3 C.

(b) Total electric flux (\(\phi\)_Total) leaving out the surface of a sphere containing net charge Q is given by the relation,

\(\phi_{Total} = \frac Q{\epsilon_0}\)

Here,

ϵ₀​ = permittivity of free space = 8.854 x 10^–12 N^–1 C² m^–2

Electric flux = 1.63 x 10⁸ N C^–1 m²

Therefore, the total electric flux leaving the surface of the sphere is 1.63 × 10⁸ N C^–1 m²

23. An infinite line charge produces a field of 9 × 10⁴ N/C at a distance of 2 cm. Calculate the linear charge density.

Answer:

Electric field produced by the infinite line charges at a distance d having linear charge density λ is given by the relation,

\(E = \frac{\lambda}{2\pi\epsilon_0d}\)

\(\lambda = 2\pi\epsilon_0dE\)

Here,

d = 2 cm = 0.02 m

E = 9 x 10⁴ N/C

ε₀ = Permittivity of free space and \(\frac 1 {4\pi\epsilon_0} = 9 \times 10^9 Nm^2C^{-2}\)

\(\lambda = \frac{0.02\times9\times10^4}{2\times9\times10^9}\)

\(\lambda = 10 \mu C/m\)

24. Two large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 17.0 × 10^–22 C /m²

What is E:

 (a) In the outer region of the first plate,

(b) In the outer region of the second plate, and ( c ) between the plates?

Answer:

The situation given in the question can be depicted through the following figure.

Parallel plates A and B are placed close to each other as shown in the figure. The region between plates A and B is labelled as II, and outer region of plate A is labelled as I, outer region of plate B is labelled as III.

The charge density of plate A can be calculated as,

σ = 17.0 × 10^–22 C/m²

Similarly, the charge density of plate B can be calculated as,

σ = – 17.0 × 10^–22 C/m²

In regions, I and III, electric field E is zero. This is because the charge is not enclosed by the respective plates.

Electric field E in region II is given by the relation,

\(E = \frac{\sigma}{\epsilon_0}\)

here,

ε₀ = Permittivity of free space = 8.854 × 10^–12 N^–1 C² m^–2

^{\(E = \frac{17.0\times10^{-22}}{8.854\times10^{-12}}= 1.92 \times10^{-10}N/C\)}

Therefore, electric field between the plates is 1.92 x 10^–10 N/C

25. An oil drop of 12 excess electrons is held stationary under a constant electric field of 2.55 × 10⁴ NC^–1 (Millikan’s oil drop experiment). The density of the oil is 1.26 g cm^–3. Estimate the radius of the drop.
(g = 9.81 m s^–2; e = 1.60 × 10^–19 C).

Answer:

Excess electron on an oil drop, n = 12

Electric field intensity, E= 2.55 × 10⁴ NC^–1

Density of oil, ρ = 1.26 g cm^–3

Acceleration due to gravity, g = 9.81 m s^–2

Charge of an electron, e = 1.60 × 10^–19 C

Radius of the oil drop = r

Force due to the electric field will be equal to the weight of the oil drop (W)

F = W

Eq = mg

Here,

q = ne = net charge on the oil drop

m = mass of the oil drop

= volume of the oil drop x density of the oil

= (4/3)πr³ x ρ

Ene= (4/3)πr³ x ρ x g

\(r = \sqrt[3]{\frac{3Ene}{4\pi pg}}\)

\(r = \sqrt[3]{\frac{3\times2.55\times10^4\times12\times1.6\times1.6\times10^{-19}}{4\times3.14\times1.26\times10^3\times9.81}}\)

\(= \sqrt[3]{946.09\times10^{-21}}\)

= 9.82 x 10^-7 m

The radius of the oil drop is 9.82 x 10^-7 m

Answer 5

 26. Which among the curves shown in Fig. cannot possibly represent electrostatic field lines?

Answer:

(a) The field lines are not normal to the surface of the conductor. Therefore, it does not represent electrostatic field lines.

(b) In this figure, the field lines are emerging from the negative charge and terminating at the positive charge. This is not possible. Therefore, it does not represent electrostatic field lines.

(c) The electric field is emerging from the positive charge and it repels each other. Therefore, the field lines shown represent electrostatic field lines.

(d) The field lines shown intersect each other. Therefore, the field lines shown does not represent electrostatic field lines.

(e) The closed loops are formed in the area between the field lines. Therefore, it does not represent electrostatic field lines.

27. In a certain region of space, electric field is along the z-direction throughout. The magnitude of electric field is, however, not constant but increases uniformly along the positive z-direction, at the rate of 10⁵ NC^–1 per metre. What are the force and torque experienced by a system having a total dipole moment equal to 10^–7 cm in the negative z-direction?

Answer:

Total dipole moment of the system, p=q × dl =−10⁻⁷ cm

The rate of increase of the magnitude of the electric field along the positive z-direction = 10⁵ NC^–1 per metre.

The force experienced by the system is given as

F = qE

F = q(dE/dl) x dl

= p x (dE/dl)

= −10⁻⁷ × 10⁻⁵

= −10⁻² N

The force experienced by the system is – 10⁻² N. This is in the negative z-direction and opposite to the direction of the electric field. Therefore, the angle between the dipole moment and the electric field is 180^o.

Torque (τ) = pE sin180^o = 0

Therefore, the torque experienced by the system is zero.

28. (a) A conductor A with a cavity as shown in Fig. (a) is given a charge Q. Show that the entire charge must appear on the outer surface of the conductor. 

(b) Another conductor B with charge q is inserted into the cavity keeping B insulated from A. Show that the total charge on the outside surface of A is Q + q [Fig. (b)]. 

(c) A sensitive instrument is to be shielded from the strong electrostatic fields in its environment. Suggest a possible way.

Answer:

(a) A Gaussian surface is considered within the conductor that encloses the cavity. Within the conductor, the electric field intensity (E) will be zero.

The charge inside the conductor is q and the permittivity of free space is ∈

According to Gauss’s law,

Flux, ϕ=E.ds = q/∈₀ 

Electric field intensity, E = 0

q/∈₀ = 0

The permittivity of the free space, ∈₀ ≠ 0

Therefore, the charge inside the conductor, q = 0

Therefore, the entire charge appears on the outer surface of the conductor.

(b) A conductor B is kept in the cavity and it is insulated from A. Hence a charge – q will be induced in the inner surface of conductor A, this in turn will induce a charge +q on the outer surface of A.  Therefore, the total charge on the outer surface of A will be equal to Q + q.

(c) The sensitive instrument can be shielded from the electrostatic field by keeping it fully enclosed inside a metallic surface.

29. A hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is \(\frac{\sigma}{2\epsilon_0}\hat n\), where \(\hat n\) is the unit vector in the outward normal direction and σ is the surface charge density near the hole.

Answer:

Considering that the hole is filled up, then the electric field intensity at a point close to the surface of the conductor is given by Gauss’s law,

Flux, \(\phi=E.ds = \frac q{\epsilon_0}\)

q = σ x ds

here, σ is the surface charge density

ds is the area of the Gaussian surface

Eds = (σ x ds)/∈₀

E = σ /∈₀

_{\(= \frac{\sigma}{\epsilon_0}\hat n\)}

Let E₁ be the electric field due to the hole and E₂ is the electric field due to the rest of the conductor. E₁ and E₂ are opposite in direction and equal in magnitude since the total electric field inside the conductor is zero.

|E₁| = |E_2​| ........(1)

The electric field E = σ /∈₀ is due to the superposition of E₁ and E₂.

E = E₁ + E₂

From equation (1) we know E₁ = E₂

Therefore, E = 2E₁

E₁ = E/2

Therefore, the electric field in the hole

\(E_1 = \frac{\sigma}{2\epsilon_0}\hat n\)

30. Obtain the formula for the electric field due to a long thin wire of uniform linear charge density E without using Gauss’s law. [Use Coulomb’s law directly and evaluate the necessary integral.]

Answer:

 

Consider a long thin wire of uniform linear charge density λ. A point P is taken at a distance r from the midpoint C of the wire.

The electric field at a point P due to the wire is E.

Let E be the electric field at point P due to the wire

Consider a small length element dx on the wire with centre O, such that OC = x

Let q be the charge on this element, q = λdx

The electric field at A due to the element is

\(dE = \frac1{4\pi\epsilon_0}\frac{\lambda dx}{OP^2}\)

OP² = r² + x²

\(dE = \frac1{4\pi\epsilon_0}\frac{\lambda dx}{(r^2 + x^2)}\)

The electric field (dE) is resolved into two rectangular components dEcosθ and dEsinθ . dEsinθ is the parallel component and dEcosθ is the perpendicular component. When the whole wire is considered, the parallel component dEsinθ is cancelled. Only the perpendicular component dEcosθ affects point P.

\(de = \frac1{4\pi \epsilon_0}\frac{\lambda dx\,cos\theta}{(r^2 + x^2)} \) .......(1)

In the ΔOCP, x = r tanθ .......(2)

Differentiating equation (1) we get

dx = rsec²θdθ

r² + x² = r² + r² tan²θ

= r² (1+ tan²θ)  = r² sec²θ

Substituting in (1)

\(dE = \frac{\lambda r\,sec^2\theta}{4\pi \epsilon_0r^2\,sec^2\theta}cos\theta\)

\(dE = \frac{\lambda}{4\pi\epsilon_0r} cos\theta d\epsilon\theta\)

The wire is of infinite length. Therefore, A and B are infinite distance apart. Therefore, θ varies from -π/2 to +π/2. The electric field intensity at the point P due to the whole wire is

\(E = \int^{\pi/2}_{-\pi/2}\,\frac{\lambda}{4\pi\epsilon_0r}cos\theta d\theta\)

\(= \frac{\lambda}{4\pi\epsilon_0r}[sin\theta]^{\pi/2}_{-\pi/2}= \frac{\lambda}{2\pi\epsilon_0r}\)

Answer 6

31. It is now established that protons and neutrons (which constitute nuclei of ordinary matter) are themselves built out of more elementary units called quarks. A proton and a neutron consist of three quarks each. Two types of quarks, the so called ‘up’ quark (denoted by u) of charge + (2/3) e, and the ‘down’ quark (denoted by d) of charge (–1/3) e, together with electrons build up ordinary matter. (Quarks of other types have also been found which give rise to different unusual varieties of matter.) Suggest a possible quark composition of a proton and neutron.

Answer:

The net charge of a proton is +e. The value +e can be got when p = \(\left(\frac23+ \frac23- \frac13\right)e\)

Therefore, the proton has two up quark and one down quark

The net charge of a neutron is 0. This can be got when n = \(\left(\frac23-\frac13-\frac13\right)e =0\) 

Therefore, the neutron has one up quark and two down quark.

32. (a) Consider an arbitrary electrostatic field configuration. A small test charge is placed at a null point (i.e., where E = 0) of the configuration. Show that the equilibrium of the test charge is necessarily unstable.

(b) Verify this result for the simple configuration of two charges of the same magnitude and sign placed a certain distance apart.

Answer:

(a) Let us consider that the equilibrium is stable. Then if the test charge is displaced in any direction it will experience a restoring force towards the null-point. This means that all the field lines near the null point will be directed towards the null point. That is, there is a net inward flux of electric field through a closed surface around the null-point. But according to Gauss’s law, the flux of electric field through a surface, not enclosing any charge, must be zero. Hence, equilibrium cannot be stable.

(b) The null-point is the mid-point of the line joining the two charges. If the test charge is displaced along the line from the null-point there is a restoring force. If the test charge is displaced normal to the line the net force takes it away from the null-point. Hence for the stability of equilibrium needs restoring force in all directions.

33. Suppose that the particle in Exercise 1.33 is an electron projected with velocity v_x = 2.0 × 10⁶ m s^–1. If E between the plates separated by 0.5 cm is 9.1 × 10² N/C, where will the electron strike the upper plate? (|e|=1.6 × 10^–19 C, m_e = 9.1 × 10^–31 kg.)

Answer:

Velocity of the electron, v_x = 2.0 × 10⁶ m s^–1

Separation between the plates, d = 0.5 cm = 0.5 x 10^-2 m

Electric field between the plates = 9.1 × 10² N/C

\(s =\frac12\left(\frac{qE}{m}\right)\left(\frac L{v_x}\right)^2\)

The distance at which the electron strike the upper plate is

\(L = \sqrt{\frac{2dmv^2_x}{qE}}\)

\(L = \sqrt{\frac{2\times0.005\times9.1\times10^{-31}\times(2\times10^6)^2}{1.6 \times10^{-19}\times9.1\times10^2}}\)

\(= \sqrt{\frac{0.364\times10^{-19}}{14.56\times10^{-17}}}\)

= 1.6 cm

The electron strikes the upper plate after 1.6 c